North Atlantic geoid high, volcanism and glaciations

Eugenio Carminati & Carlo Doglioni

Dipartimento di Scienze della Terra, Università La Sapienza, Roma, Italy

eugenio.carminati@uniroma1.it & carlo.doglioni@uniroma1.it

Summary

Shallow topography, a geoid high and intense volcanism on the northern Mid Atlantic Ridge are interpreted as enhanced by the loading on the adjacent continents by ice caps during upper Cenozoic glaciations. The load of ice packs on the continental lithospheres of North America and northern Europe generated radial mantle flow at depth. In our model, these currents, where flowing from west and east, faced each other below the northern Atlantic, joining together and upwelling. Numerical modeling of this process supports the development of dynamic topography leading to uplift of the sea-floor and inducing a regional geoid high. The upper mantle rises to a few kilometres shallower than average, partly as a result of being mechanically lifted by the lower mantle (Figure 1). This may have contributed to greater asthenospheric melting, and to ridge centered excess magmatism, as observed in the Northern Atlantic.

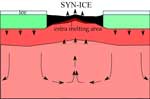
Figure 1. The tilted car simulates the uplift mechanism of the upper mantle in the North Atlantic.

1. Introduction

The lithosphere generated by the mid-Atlantic Ridge (MAR) east of Greenland underlies the youngest (< 60 Ma) and narrowest part of the Atlantic Ocean. This portion of the northern Atlantic shows three peculiar characteristics:

1. It is about 1-3 km shallower than the average mid-oceanic ridge (Figure 2a, b);
2. It displays broad positive gravity (> 30 mGal) and geoid (> 50 m) anomalies (Figure 2c, d, f);
3. It is the locus of anomalously great magmatic productivity, resulting in the thickest oceanic crust found along the entire MAR, being up to about 40 km thick beneath Iceland (Kaban et al., 2002). The thickness of the Cretaceous-Early Cenozoic (pre-glaciations) oceanic crust in the northern Atlantic is 4-6 km in average (e.g., Shillington et al., 2006).

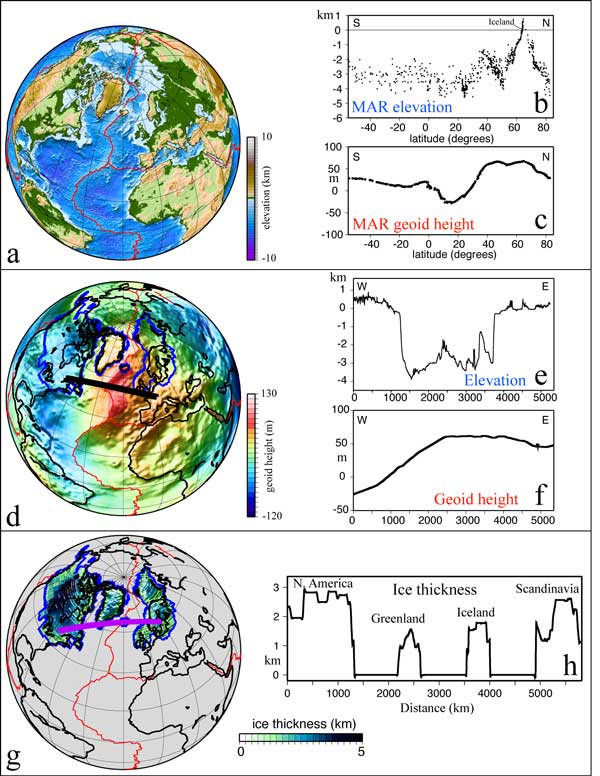
Figure 2: a) Topography (data after ETOPO1); b) Elevation of the MAR. The bathymetry along the MAR shows a high in the northern Atlantic which is limited not only to the Iceland area but it extends ca 20° northward and 40° southward; c) Geoid anomaly along the MAR (data after the EGM96 model); d) Geoid height. The north Atlantic geoid high is located between the North American and Scandinavian ice bodies; e) topography-bathymetry along the cross-section on the map at left; f) geoid height along the same section; The blue curves in panel d) show the borders of the ice bodies according to ICE-5G. The geoid is shallower along the eastern flank of MAR and the crest of the anomaly is offset to the east of the oceanic ridge. g) Thickness in map, and h) cross-section in purple of the ice cap at the last glacial maximum (21 ka BP; data after the ICE-5G model; Peltier, 2004). Mid-ocean ridges are shown as red lines. The purple great circle in panel g) shows the trace of the modeled profile of Figure 4.

Several papers attribute these features to a mantle plume (e.g., Vink, 1984). However, the deep plume hypothesis has been questioned on many grounds (e.g., Foulger & Anderson, 2005) including the persistence of magmatism on the westerly moving ridge, giving trails of volcanic productivity both west and east of Iceland, the absence of evidence for high temperature, and the presence of hydrous mantle lowering the melting point (Bonatti, 1990). There is also no evidence from seismic tomography for a plume in the lower mantle (Foulger et al., 2001; Ritsema & Allen, 2003). Moreover, the Icelandic geochemical signature is not restricted to Iceland, but continues both north and south along the MAR (Taylor et al., 1997).

Here we test numerically a model in which far field superficial loading of the mantle by the ice caps in North America and northern Europe can contribute to the anomalous features of the North Atlantic (Figure 3), i.e., uplift of the north Atlantic mantle, the geoid anomaly and a higher degree of melting due to faster adiabatic decompression induced by mantle upwelling.

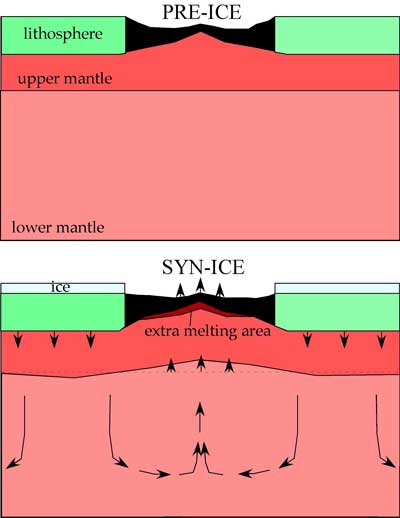
Figure 3: Model of the North Atlantic uplift generated by the loading of the ice caps over the adjacent continents. Melting occurs in the asthenosphere, but it is facilitated by the upward pumping of the deeper mantle. Hysteresis is required in order to maintain the shallower depleted asthenosphere after melting.

The magmatic overproduction predicted by this model represents another type of volcanism able to generate trails, complementary to shear heating (Doglioni et al., 2005; Anderson, 2010), extra water in the mantle (Bonatti, 1990), and migrating rifts. This provides another explanation for the excess magmatism commonly attributed to plumes, illustrating that such magmatism can have many origins, and reinforcing evidence for their shallow nature.

2. Model description and results

Assuming Earth is a uniform viscous half-space, with a cylindrical ice-load, it can be shown analytically (e.g., Cathles, 1975) that the depth at which the vertical displacement induced by ice loading/unloading is 0.5, 0.2 or 0.1 x the surface value, and equal to 1.4R0, 2.5R0 and 3.3R0 (where R0 is the radius of the cylinder; i.e., 825 km, 1474 km and 1815 km for the Fennoscandian ice sheet, which has R0=550 km). Numerical solutions have also shown that the ice cycles in the Canadian region induced vertical motions exceeding 60° (6600 km) from the ice center (e.g., Cathles, 1975).

Here we test the combined effects of the glacial cycles in North America and Europe on regional mantle flow. The aim of our finite element modeling, performed using COMSOL 3.5 software, is to evaluate the velocity field induced within the upper mantle by glaciation cycles rather than to reproduce exactly the surface velocities. This allowed us to adopt some major simplifying assumptions, such as two-dimensionality (2D), neglecting the load due to water redistribution during the ice formation and melting, and using a simplified ice model.

The model adopts a 2D plane strain approximation and includes lithosphere, upper and lower mantle (Figure 4). All the layers are described by a compressible linear viscoelastic (Maxwell) rheology. The assumed elastic constants and viscosities are listed in Table I. The elastic structure is consistent with the PREM model (Dziewonski & Anderson, 1981) and the viscosities are consistent with values normally used for glacial isostatic rebound modeling (e.g., Mitrovica & Peltier, 1993; Kaufmann & Lambeck, 2002). Gravity acceleration and density vary with depth according to the PREM model. Gravity is applied as a body force and the ice load as a boundary condition. Ice thickness varies with time but is kept laterally constant for each area. The model is run from 150 ka BP to the present. The ice thickness is kept at zero between 150 ka and 120 ka BP and is then linearly increased to reach the maximum thickness at 105 ka BP. It is then kept constant until 21 Ka BP. Between 21 Ka and 6 Ka BP the ice thickness is linearly decreased to zero, with the exception of Greenland, where it is decreased to 750 m. The maximum thicknesses is assumed to vary regionally–2500 m for North America, 1300 m for Greenland, 2000 m for Scandinavia and 2000 m for Iceland (when applied). Such values are consistent with Figure 2h, which shows maximum ice thicknesses along the trace of the modeled section at 21 ka BP according to the ICE-5G model (Peltier, 2004).

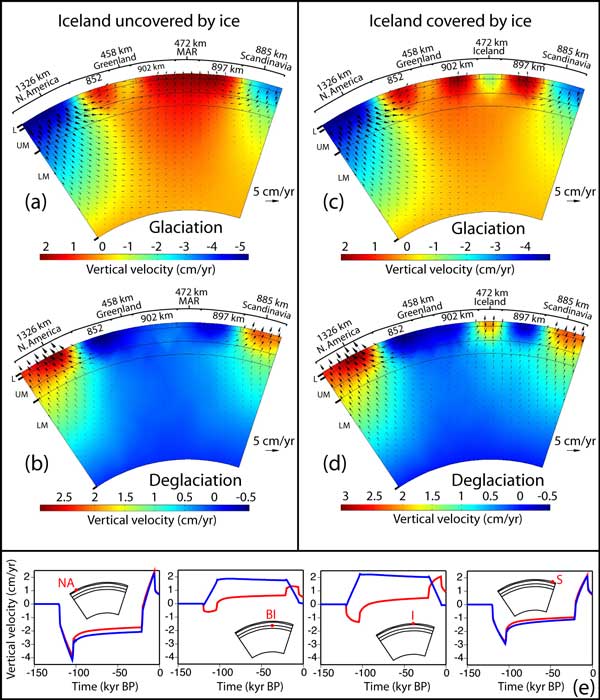
Figure 4: Vertical velocities and velocity fields predicted by the models immediately after the formation of the ice caps (105 ka BP, panels a and c) and soon after their melting (6 ka BP, panels b and d). Panels a and b refer to a model without ice during the glacial period in the Iceland region, and panels c and d to a model with Iceland affected by ice. Panel e shows the vertical rates through time at four different locations marked by the red dots (NA, North America; BI, beneath Iceland; I, Iceland; S, Scandinavia). Blue line is with Iceland unaffected by ice, red line represents the case of Iceland covered by ice. Click here or on figure for enlargement.

Table 1: Elastic and viscous parameters used in the calculations.

The bottom of the model is fixed normally to the boundary and is free to slip tangentially. Symmetry conditions are imposed on the left and right boundaries. This is reasonable since the tips of the modeled section are located approximately at the center of the American and Scandinavian ice masses. The model surface is left free in areas unaffected by ice formation. We use a set of ca. 3800 triangular elements. Modeling results are shown for the time steps of 105 ka and 6 ka BP, representative of the glaciation and deglaciation scenarios respectively. Although no constraints are available for past mantle velocity simulations, we are confident that the patterns and the order of magnitude of the calculated velocities are realistic. This confidence is justified by the positive match between simulated and observed present-day vertical velocities for well-constrained areas such as Scandinavia.

Two scenarios are modeled. In the first Iceland is covered by ice during the glaciation, while in the second Iceland is assumed to be ice-free. The first model simulates the evolution in the transect of Figure 2, while the second simulates a section just north or south of Iceland. Figure 4 shows the vertical velocities and the velocity field predicted for the two scenarios. Both scenarios indicate a convergence of velocity vectors towards the Atlantic during formation of the ice cap, with a prevalence of horizontal directions of motion. Below Iceland and the surrounding Atlantic the velocity vectors turn vertical with a general upwelling (rates of up to 2 cm/a in the Iceland ice-free scenario). In the Iceland-covered scenario, the upwelling is limited to the Atlantic region with rates of less than 2 cm/a. Below Iceland the lowermost upper mantle moves upward slowly (< 0.5 cm/a), while the shallower upper mantle moves downward, due to the Icelandic ice load. During the same glaciation period, a downward flow at rates of 2/4 cm/a is predicted for North America and Scandinavia.

The velocity field is reversed during deglaciation, with the mantle flowing downward and away from the central Atlantic region and upward below Scandinavia and North America. Figure 4e shows that development of the velocity field associated to glaciation and its reversal during deglaciation is fast, due to the elastic component of rheology. Present-day rates, although with lower magnitudes, show for the two scenarios velocity patterns similar to those of Figure 4b and 4d. Thus the dynamic topography attained during the glaciation period has not been completely recovered, due to the viscous component of the lithosphere and mantle rheology.

The models show that the ice load induces an upward flow below the MAR generating dynamic topography consistent with the geoid high measured in the region. The results of the model that assumes Iceland free of ice allowed us to predict, at 21 ka BP (i.e., just before the beginning of deglaciation), a geoid anomaly of ca. 70 m for the center of the Atlantic ocean (location I in Figure 4e). The geoid anomaly was calculated as:

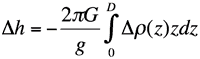
(Turcotte & Schubert, 2002), where Dh is the geoid anomaly, g is the gravity acceleration, Dr(z) is the anomalous density at depth z, D is the compensation depth (chosen as the bottom of our model) and G is the Newtonian constant (6.67x10¹¹ m³ kg^-1 m^-2). Although this calculation is just a rough estimate, since it includes only the upward motion below the MAR and does not include crust formation, mantle partial melting and other thermal processes, it is compatible with the present day anomaly of the region (ca. 60 m; Figure 2), showing that present-day geoid anomaly and high topography of the region are remnants of the glaciation.

Moreover, mantle upwelling may enhance mantle partial melting and explain, at least in part, the anomalously intense magmatic activity of the region. Assuming an average 7-10% melt of the asthenosphere (e.g., Langmuir & Forsyth, 2007) under the northern MAR, the cumulative uplift of ca. 2 km of the mantle during the glaciations would increase the melting by a few percent (depending on water content, initial mantle composition and temperature, spreading rate, etc.), producing a larger volume of magma delivered to the surface.

3. Discussion and conclusions

Ice loading/unloading can have a regional impact on mantle flow velocities (Figure 3). The MAR shallow bathymetry (Figure 2) and the geoid regional positive anomaly of the northern Atlantic (Tapley et al., 2005) are in an area intermediate between the ice caps in north America and Europe during the last glaciation. Moreover, the same area is occupied by the largest volcanic province in the northern Atlantic. If our model is correct, we speculate a glacio-eustatic Milankovitch periodicity in north Atlantic magma production.

The oldest rocks in Iceland are ~ 15 Ma old (Hardarson et al., 1997). The same authors noted chemical variations of basalts, generated by a variably depleted mantle. Iceland possibly emerged at that time or later, and it experienced ice loading as well. The time of the onset of glaciations in the northern hemisphere is still debated. It has been shown how the onset of glaciations in the northern hemisphere is older (Eocene-Oligocene) than previously estimated (Eldrett et al., 2007). Recent deep sea drilling provided evidence for a middle Eocene initiation of the icehouse of the Arctic area (Moran et al., 2006).

Although we modeled a single ice cycle, the productivity of magma over geological periods is expected to be influenced by the superposition of several ice cycles on the process of oceanic spreading. The remote loading of ice can determine an upwelling of the mantle elsewhere, generating larger volumes of melt due to mantle adiabatic decompression below the ridge. Vice versa, the ice load in a volcanic area (e.g., along the MAR in Iceland) can locally buffer eruption, tuning the frequency of magmatic delivery, and generating a lower degree of melting and a longer residence time of melts in the mantle. Our model predicts a relatively low intensity of magmatism along the northern segment of the MAR during the present interglacial period.

We note that the North Atlantic geoid height is presently decreasing, while it is increasing on the adjacent continental areas, as shown by the Grace project data (e.g., Tapley et al., 2004). The decrease of the geoid has been related to the melting of ice in Greenland (Ramillien et al., 2006), but it could also be related also to decreasing upwelling beneath the northern MAR due to the absence of ice caps on the continents. Conversely, continental areas show an increase of the geoid because the mantle is rising, recovering the subsidence previously generated by the ice loading. When mantle rises and melts beneath a ridge, it becomes lighter (Oxburgh & Parmentier, 1977). Therefore the process is possibly not entirely reversible since the uplifted and depleted mantle cannot be re-downwelled to its original position, by the down-flow motion induced by deglaciation, because of the permanent increase in buoyancy following melting.

During the time frame considered (say the last 20-30 Ma) we may expect about 180-250 oscillations associated with the eccentricity of the Earth’s orbit, or more than twice as many in the case of obliquity related cycles. The model presented rather shows the effects of only one single cycle of loading and unloading. Assuming an irreversible component on each cycle, the present geoid high would represent the sum of the all episodes, a sort of vibration-generating hysteresis in the uplift of the mantle.

In summary, we suggest that the ice caps on the continents of the northern hemisphere generated flow in the underlying mantle that converges in the northern Atlantic from west and east, upwelling along the northern MAR. The eastward offset of the geoid high relative to the MAR could be due to a larger ice load on the northern American continent, although we cannot neglect a contribution from the relative eastward mantle flow implicit in the notion of the westward drift of the lithosphere (Crespi et al., 2007), able to generate an asymmetry of ocean ridges worldwide (Doglioni et al., 2003; 2007; Panza et al., 2010) and possibly contributing to the vertical motion of continents (Carminati et al., 2009). This model implies that the over production of magmatism in the northern Atlantic could be sourced from shallow depth in the asthenosphere, where mantle rises as a result of pumping from deeper mantle flow.

References

• Anderson, D.L., 2010, Hawaii, Boundary Layers and Ambient Mantle--Geophysical Constraints, Journal of Petrology, doi: 10.1093/petrology/egq068.
• Bonatti, E. (1990), Not So Hot "Hot Spots" in the Oceanic Mantle, Science, 250, 107-111.
• Carminati E., Cuffaro M & Doglioni C. (2009), Cenozoic uplift of Europe. Tectonics, 28, TC4016, doi:10.1029/2009TC002472.
• Carminati E. and Doglioni C. (2010), North Atlantic geoid high, volcanism and glaciations. Geophys. Res. Lett., 37, L03302, doi:10.1029/2009GL041663.
• Cathles, L.M. (1975), The viscosity of the Earth's mantle Princeton, New Jersey, Princeton University Press, 386 pp.
• Crespi, M., Cuffaro, M., Doglioni, C., Giannone, F., and Riguzzi, F. (2007), Space geodesy validation of the global lithospheric flow. Geophys. J. Int., 168, 491-506, doi: 10.1111/j.1365-246X.2006.03226.x.
• Doglioni, C., E. Carminati, and E. Bonatti (2003), Rift asymmetry and continental uplift, Tectonics, 22, 1024, doi:10.1029/2002TC001459.
• Doglioni C., Carminati E., Cuffaro M. and Scrocca D. (2007), Subduction kinematics and dynamic constraints. Earth Science Reviews, 83, 125-175, doi:10.1016/j.earscirev.2007.04.001.
• Doglioni C., Green D. and Mongelli F. (2005), On the shallow origin of hotspots and the westward drift of the lithosphere: in Plates, Plumes and Paradigms, G.R. Foulger, J.H. Natland, D.C. Presnall, and D.L. Anderson (Eds), GSA Sp. Paper 388, 735-749.
• Dziewonski, A. M., and D. L. Anderson (1981), Preliminary reference Earth model, Phys. Earth Planet. Int., 25, 297–356.
• Eldrett, J. S., I. C. Harding, P. A. Wilson, E. Butler, and A. P. Roberts (2007), Continental ice in Greenland during the Eocene and Oligocene, Nature, 446, 176-179.
• Foulger, G. R., and D. L. Anderson (2005), A cool model for the Iceland hotspot, J. Volc. Geother. Res., 141, 1– 22.
• Foulger, G. R., et al. (2001), Seismic tomography shows that upwelling beneath Iceland is confined to the upper mantle, Geophys. J. Int., 146, 504–530.
• Gripp, A. E., and R. G. Gordon (2002), Young tracks of hotspots and current plate Velocities, Geophys. J. Int., 150, 321-361.
• Hardarson, B. S., J. G. Fitton, R. M. Ellam, M. S. Pringle (1997), Rift relocation - a geochemical and geochronological investigation of a palaeo-rift in northwest Iceland, Earth Planet. Sci. Lett., 153, 181-196.
• Kaban, M. K., O. G. Flóvenz, and G. Pálmason (2002), Nature of the crust-mantle transition zone and the thermal state of the upper mantle beneath Iceland from gravity modelling, Geophys. J. Int., 149, 281-299.
• Kaufmann, G., and K. Lambeck (2002), Glacial isostatic adjustment and the radial viscosity profile from inverse modeling, J. Geophys. Res., 107, 2280. doi:10.1029/2001JB000941.
• Langmuir, C. H. and D. H. Forsyth (2007), Mantle Melting Beneath Mid-Ocean ridges, Oceanography, 20, 1, 78-87.
• Mitrovica, J. X., and W. R. Peltier (1993), Constraints on mantle viscosity from relative sea level variations in Hudson Bay, Geophys. J. Int., 19, 1185-1188.
• Molnar, P., and J. Stock (1987), Relative motions of hotspots in the Pacific, Atlantic, and Indian oceans since Late Cretaceous time, Nature, 327, 587-591.
• Moran, K. et al. (2006), The Cenozoic palaeoenvironment of the Arctic Ocean, Nature, 441, doi:10.1038/nature04800.
• Oxburgh, E. R., and E. M. Parmentier (1977), Compositional and density stratification in oceanic lithosphere; causes and consequences, J. Geol. Soc. London, 133/4, 343-355.
• Panza, G., C. Doglioni, and A. Levshin (2010), Asymmetric ocean basins, Geology, 38, 59–62.
• Peltier, W. R. (2004), Global glacial isostasy and the surface of the ice-age Earth: the ICE-5G (VM2) model and GRACE, Ann. Rev. Earth Planet. Sci., 32, 111-149.
• Ramillien, G., A. Lombard, A. Cazenave, E. R. Ivins, M. Llubes, F. Remy, and R. Biancale (2006), Interannual variations of the mass balance of the Antarctica and Greenland ice sheets from GRACE, Glob. Planet. Change, 53, 198-208.
• Ritsema, J., and R. M. Allen (2003). The elusive mantle plume, Earth Planet. Sci. Lett., 207, 1-12.
• Shillington, D. J., W. S. Holbrook, H. J. A. Van Avendonk, B. E. Tucholke, J. R. Hopper, K. E. Louden, H. C. Larsen, G. T. Nunes (2006), Evidence for asymmetric nonvolcanic rifting and slow incipient oceanic accretion from seismic reflection data on the Newfoundland margin, J. Geophys. Res., 111, B09402, doi:10.1029/2005JB003981.
• Tapley, B. D., S. Bettadpur, J. Ries, P. F. Thompson, M. M. Watkins (2004), GRACE Measurements of Mass Variability in the Earth System, Science, 305, 5683, 503-505.
• Tapley, B. et al. (2005), GGM02 – An improved Earth gravity field model from GRACE, J. Geod., 79, 467–478.
• Taylor, R. N., M. F. Thirlwall, B. J. Murton, D. R. Hilton, and M. A. M. Gee (1997), Isotopic constraints on the influence of the Icelandic plume, Earth Planet. Sci. Lett., 148, El -E8.
• Turcotte, D. L., and G. Schubert (2002), Geodynamics. Cambridge University Press, 456 pp.
• Vink, G. E. (1984), A hotspot model for Iceland and the Voring plateau, J. Geophys. Res., 89, 9949-9959.